Crystallinity and semicrystalline polymers
The driving force behind crystallization is best explained in a thermodynamic context. A polymer will crystallize in case it can form sufficiently strong and frequent intermolecular attractions to compensate for the loss in disorder in the phase transition from disordered to ordered. It is a primary phase transition going from liquid to solid. The change in Gibbs free energy, ∆G, is expressed as:
∆G = ∆H-T∆S
The heat of crystallization, ∆H, which is exothermal, thereby renders a negative value. Also the change of ∆S, where S is a measure of the systems disorder, is negative since crystallization makes the system less disordered. Hence, ∆H has to compensate for the loss of ∆S at a certain crystallization temperature in order to reduce Gibbs free energy. The lower the crystallization temperature, T, the smaller the term -T∆S becomes and the potential to reduce Gibbs energy increases. During heating melting of the crystalline structure will occur at a temperature where ∆G equals zero. This constitutes its melting temperature Tm = ∆H/∆S. For polymers with strong and dense intermolecular attraction or for polymers that are rather rigid, thereby do not lose quite as much disorder during their transition they get high Tm’s. For rigid polymers that form strong and dense intermolecular attraction the Tm becomes very high; typical of high performance polymers. It is also that there is a substantial margin between the Tm and Tg to enable the necessary polymer diffusion for crystallization to take place. As a rule of thumb the highest crystallization rate is accomplished right between the Tm and Tg, i.e. (Tm+Tg)/2.
A crystalline structure can be described by an ordered 3D structure where, in this case the polymer has arranged itself. The smallest common denominator can be described as a unit cell usually in the form of a cubic, rhombic or distorted rhombic configuration. They take on the configuration that enables the most intermolecular bonds. This may be associated with all-trans or perhaps a helix- conformation. Depending on the degree of supercooling and the cooling-rate during crystallization they will form lamellas of varying folding length. As illustrated in Figure 8 the ordered sequences are linked to amorphous sequences. A polymer can never be completely crystalline, hence they are called semicrystalline. Since the polymer may start to crystallize at more than one spot at once, their diffusion will be restricted.
Even if very high degree of crystallinity can be accomplished upon precipitation of a polymer solution they always contain end groups that affect the crystallization. This is also the case upon crystallization from the molten state. Hence, polymers with a lot of end-groups, which is found for low molar mass linear and for branched polymers renders low degree of crystallinity. Also in cases of very high molar mass polymers the number of entanglements will reduce polymer diffusion needed to accomplish high the degree of crystallinity. Hence there is an optimum in terms of molar mass and thermal history for each polymer.
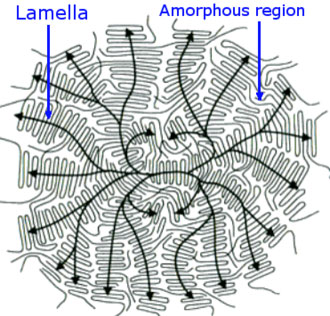
Figure 8. A schematic spherulite of a semicrystalline polymer. Arrows indicate where it was nucleated and how it grew.
Materialscientist at Wikimedia / CC BY-SA 3.0
Task: How can you easily determine whether a polymeric material is semicrystalline or amorphous?
It floats in water.
It is really brittle.
Its mix of crystalline and amorphous domains makes it opaque or even white.
It is completely transparent since it’s semicrystalline.

All efforts have been made to ensure materials created by the EDU comply with current accessibility guidelines (JISC: Support for learners with disabilities).
If further assistance is required with accessibility matters please contact the student support section in your academic partner UHI: Accessing learner support.
We welcome any comments on how to improve this unit. Please feel free to pass these on at any time.
If you have any difficulty viewing this resource please contact EDU (edu@uhi.ac.uk) with:
- the name of the resource;
- a description of the problem (please give as much detail as possible);
- the section of the resource where the problem occurred;
- your internet browser (you can check your browser version at: http://detectmybrowser.com/).
UHI provides links to external sources of information and may refer to specific Web sites, products, processes or services within this resource. Such references are examples and are not endorsements and whilst every effort is taken to ensure the accuracy of information provided UHI is not responsible for any of the content or guidance. You are advised to exercise caution.
Audio
Video
Reading
Download
Information
External link
Activity
Question
Asterisk
Discussion
Collaboration
Reflection/journal/log
History
Pause for thought
Download a copy of this resource in PDF format.
You can also print individual pages by printing directly from the browser.